addition and subtraction of fraction problem solving

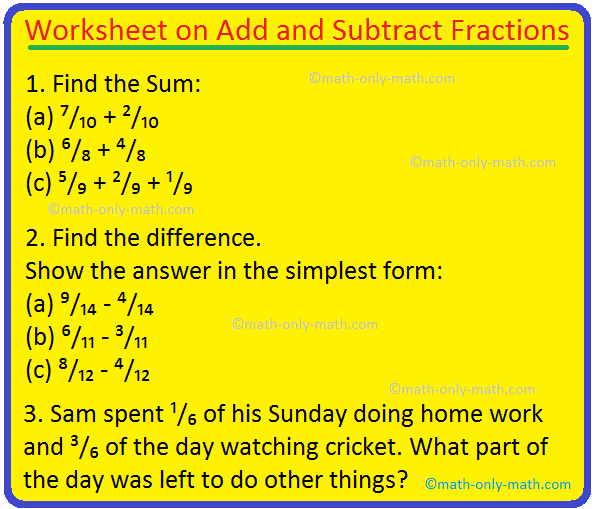
Worksheet on Add and Subtract Fractions

Recall the topic carefully and practice the questions given in the math worksheet on add and subtract fractions. The question mainly covers addition with the help of a fraction number line, subtraction with the help of a fraction number line, add the fractions with the same denominator, subtract the fractions with the same denominator and word problems on add and subtract fractions.

I. Addition and Subtraction of Like Fractions:

For adding or subtracting like fractions, we follow the following steps.

Working Rules for Addition and Subtraction of Like Fractions:

Step I:  Add or subtract the numerators of the given fractions and keep the denominator as it is.

Step II:  Reduce the fraction of its lowest term.

Step III:  If the result is an improper fraction, convert it into a mixed fraction.

sum or difference of like fractions

                  =  Sum of Difference of Numerators

                             Common denominator

II: Addition and Subtraction of Unlike Fractions:

For adding or subtracting unlike fractions, we follow these steps.

Working Rules  for Addition and Subtraction of Like Fractions:

Step I: Find the LCM of denominators of the given fractions.

Step II: Convert unlike fractions into like fractions by making LCM as their denominator.

Step III: Add or subtract the like fractions.

1.   Add with the help of a fraction number line: (a) \(\frac{2}{3}\) + \(\frac{1}{3}\)

(b) \(\frac{3}{7}\) + \(\frac{2}{7}\)

(c) \(\frac{6}{10}\) + \(\frac{1}{10}\)

2.   Subtract with the help of a fraction number line:   (a) \(\frac{9}{10}\) - \(\frac{3}{10}\)

(b) \(\frac{5}{6}\) - \(\frac{2}{6}\)

(c) \(\frac{7}{8}\) - \(\frac{4}{8}\)

3. Add:  

(a) \(\frac{7}{10}\) + \(\frac{2}{10}\)

(b) \(\frac{6}{8}\) + \(\frac{4}{8}\)

(c) \(\frac{5}{9}\) + \(\frac{2}{9}\) + \(\frac{1}{9}\)

(d) \(\frac{8}{11}\) + \(\frac{2}{11}\)

(e) \(\frac{4}{9}\) + \(\frac{7}{9}\)

(f) \(\frac{3}{8}\) + \(\frac{5}{8}\) + \(\frac{2}{8}\)

(g) \(\frac{4}{6}\) + \(\frac{2}{6}\) + \(\frac{1}{6}\)

(h) \(\frac{7}{12}\) + \(\frac{5}{12}\) + \(\frac{6}{12}\)

4. Find the difference. Remember to show the answer in the simplest form: (a) \(\frac{9}{14}\) - \(\frac{4}{14}\)

(b) \(\frac{6}{11}\) - \(\frac{3}{11}\)

(c) \(\frac{8}{12}\) - \(\frac{4}{12}\)

(d) \(\frac{12}{15}\) - \(\frac{9}{15}\)

(e) \(\frac{12}{13}\) - \(\frac{9}{13}\)

(f) \(\frac{6}{10}\) - \(\frac{2}{10}\)

(g) \(\frac{9}{16}\) - \(\frac{5}{16}\)

(h) \(\frac{12}{14}\) - \(\frac{8}{14}\)

5. Add the following fractions:

(i) \(\frac{4}{7}\) + \(\frac{5}{7}\) 

(ii) \(\frac{3}{7}\) + \(\frac{11}{7}\)

(iii) \(\frac{3}{23}\) + \(\frac{11}{23}\) + \(\frac{1}{23}\)

(iv) \(\frac{2}{5}\) + \(\frac{3}{20}\)

(v) \(\frac{2}{7}\) + \(\frac{4}{9}\)

(vi) 3\(\frac{7}{9}\) + 2\(\frac{5}{6}\) + 6\(\frac{1}{3}\)

(vii) \(\frac{2}{15}\) + \(\frac{5}{12}\) + \(\frac{4}{20}\)

(viii) \(\frac{11}{16}\) + \(\frac{3}{10}\) + \(\frac{9}{24}\)

(ix) 1\(\frac{3}{8}\) + \(\frac{5}{8}\)

(x) 4\(\frac{3}{5}\) + 2\(\frac{7}{20}\)

(xi) \(\frac{8}{15}\) + 2\(\frac{1}{15}\)

(xii) 3\(\frac{1}{5}\) + 4\(\frac{1}{10}\) + 5\(\frac{1}{15}\)

6. Subtract the following fractions:

(i) \(\frac{7}{9}\) - \(\frac{2}{9}\)

(ii) \(\frac{3}{4}\) - \(\frac{3}{4}\)

(iii) 8\(\frac{2}{9}\) - 2\(\frac{5}{9}\)

(iv) \(\frac{11}{20}\) - \(\frac{4}{15}\)

(v) \(\frac{8}{15}\) - \(\frac{1}{7}\)

(vi) 5\(\frac{1}{40}\) - \(\frac{4}{25}\)

(vii) 6\(\frac{2}{16}\) - 3\(\frac{1}{6}\)

(viii) 3\(\frac{7}{8}\)  - \(\frac{6}{7}\)

(ix) 7\(\frac{3}{21}\) - 6\(\frac{3}{28}\)

7. Simplify the following combination of addition and subtraction of fractions:

(i) \(\frac{3}{4}\) + \(\frac{2}{3}\) - \(\frac{1}{2}\)

(ii) \(\frac{3}{8}\) + \(\frac{7}{8}\) - \(\frac{1}{8}\)

(iii) \(\frac{12}{25}\) - \(\frac{3}{25}\) + \(\frac{9}{25}\)

(iv) 2\(\frac{1}{2}\)+ 3\(\frac{3}{5}\) - 1\(\frac{1}{3}\)

(v) 11\(\frac{1}{4}\) - 4\(\frac{3}{5}\) + \(\frac{8}{11}\)

(vi) \(\frac{7}{18}\) - \(\frac{1}{12}\) + \(\frac{1}{6}\)

Worksheet on Word Problems on Addition and Subtraction of Like Fractions:

8. Solve these problems: (a) \(\frac{1}{3}\) of the school garden has vegetable and another \(\frac{1}{3}\) has flowers. What part of the garden is left to grow grass? (b) Sam spent \(\frac{1}{6}\) of his Sunday doing home work and \(\frac{3}{6}\) of the day watching cricket. What part of the day was left to do other things? (c) My mother ate \(\frac{1}{8}\) of the cake and my father \(\frac{3}{8}\). How much of the cake has been eaten and how much is left? (d) Pearl bought \(\frac{2}{3}\) of her school books last week. What part is still left to be bought? (e) Sonia walked \(\frac{3}{8}\) of the distance to school and ran \(\frac{5}{8}\) of the distance. How much more of the distance does she need to cover?

(f) Emma likes chocolate. One day she bought a chocolate and ate \(\frac{5}{8}\) of it in the morning and \(\frac{2}{8}\) in the evening. How much part of the chocolate has she eaten?

(g) James and  Lucas are eating a pizza.  James ate \(\frac{3}{4}\) of the pizza and  Lucas ate  \(\frac{1}{4}\) of pizza. Who ate more pizza?

(h) Sophia completed \(\frac{2}{5}\) of her homework before going out for play. She did \(\frac{1}{5}\) of her homework after the play. How much homework did she complete altogether?

(i) David distributed \(\frac{19}{24}\) apples in his class and gave \(\frac{2}{24}\) to his friend Richard. What fraction of apples he gave away in all?

(j) Mary read \(\frac{2}{9}\) of her book in the morning and \(\frac{5}{9}\) in the evening. What fraction of the book has she read?

(k) A piece of ribbon is \(\frac{12}{15}\) m long. A piece of \(\frac{4}{15}\) m is cut from it. What is the fraction of the remaining ribbon?

(l) Nancy saves \(\frac{2}{7}\) of her salary and uses \(\frac{1}{7}\) for paying the house rent. How much salary is she left with?

Answers for the worksheet on add and subtract fractions are given below to check the exact answers of the above questions on adding & subtracting fractions. 

5.  (i) 1\(\frac{2}{7}\) 

(iii) \(\frac{15}{23}\) 

(iv) \(\frac{11}{20}\) 

(v) \(\frac{46}{63}\) 

(vi) 12\(\frac{17}{18}\)

(vii) \(\frac{45}{60}\) or, \(\frac{3}{4}\)

(viii) 1\(\frac{87}{240}\)

(x) 6\(\frac{19}{20}\)

(xi) 2\(\frac{9}{15}\)

(xii) 12\(\frac{11}{30}\)

6. (i) \(\frac{5}{9}\)

(ii) \(\frac{1}{4}\)

(iii) 5\(\frac{6}{9}\) or 5\(\frac{2}{3}\)

(iv) \(\frac{17}{60}\)

(v) \(\frac{41}{105}\)

(vi) 4\(\frac{173}{200}\)

(vii) 2\(\frac{46}{48}\) or 2\(\frac{23}{24}\)

(viii) 3\(\frac{1}{56}\)

(ix) 1\(\frac{3}{84}\) or 1\(\frac{1}{28}\)

7.  (i) \(\frac{11}{12}\)

(ii) 1\(\frac{1}{8}\)

(iii) \(\frac{18}{25}\)

(iv) 4\(\frac{23}{30}\)

(v) 7\(\frac{83}{220}\)

(vi) \(\frac{17}{36}\)

You might like these

2nd Grade Fractions Worksheet | Basic Concept of Fractions | Answers

In 2nd Grade Fractions Worksheet we will solve different types of problems on fractions, one-whole, one-half, one-third, one-fourth, three-fourth or s quarter. In a fraction, it is important that the 'whole' is divided into 'equal' parts.

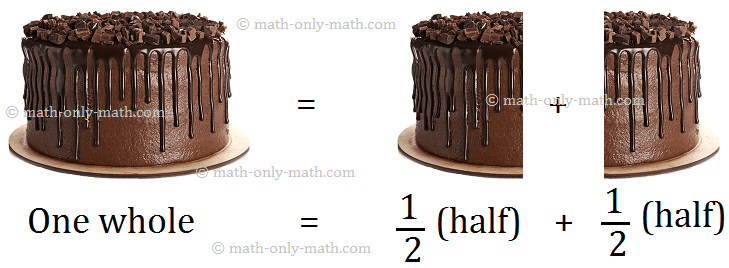
Concept of Fractions |Concept of Half| Concept of One Fourth|Two Third

Concept of fractions will help us to express different fractional parts of a whole. One-half When an article or a collection of objects is divided into two equal parts is called as half of the whole.

Worksheet on Word Problems on Fractions | Fraction Word Problems | Ans

In worksheet on word problems on fractions we will solve different types of word problems on multiplication of fractions, word problems on division of fractions etc... 1. How many one-fifths

Worksheet on Fractions | Questions on Fractions | Representation | Ans

In worksheet on fractions, all grade students can practice the questions on fractions on a whole number and also on representation of a fraction. This exercise sheet on fractions can be practiced

Conversion of Mixed Fractions into Improper Fractions |Solved Examples

To convert a mixed number into an improper fraction, we multiply the whole number by the denominator of the proper fraction and then to the product add the numerator of the fraction to get the numerator of the improper fraction. I

Verification of Equivalent Fractions | Exploring Equivalent Fractions

We will discuss here about verification of equivalent fractions. To verify that two fractions are equivalent or not, we multiply the numerator of one fraction by the denominator of the other fraction. Similarly, we multiply the denominator of one fraction by the numerator

Representations of Fractions on a Number Line | Examples | Worksheet

In representations of fractions on a number line we can show fractions on a number line. In order to represent 1/2 on the number line, draw the number line and mark a point A to represent 1.

Addition and Subtraction of Fractions | Solved Examples | Worksheet

Addition and subtraction of fractions are discussed here with examples. To add or subtract two or more fractions, proceed as under: (i) Convert the mixed fractions (if any.) or natural numbers

Fractions in Descending Order |Arranging Fractions an Descending Order

We will discuss here how to arrange the fractions in descending order. Solved examples for arranging in descending order: 1. Arrange the following fractions 5/6, 7/10, 11/20 in descending order. First we find the L.C.M. of the denominators of the fractions to make the

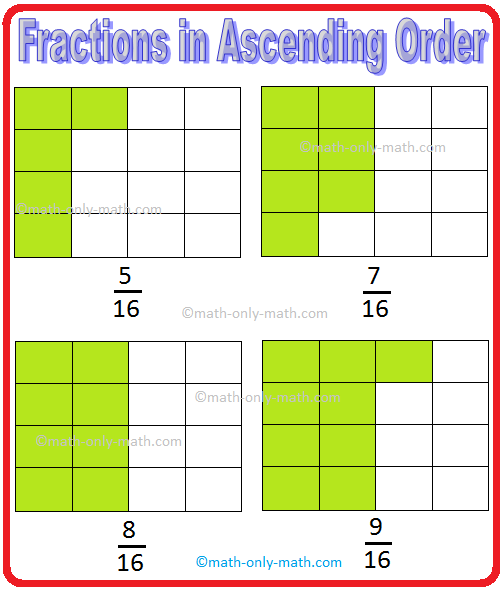
Fractions in Ascending Order | Arranging Fractions | Worksheet |Answer

We will discuss here how to arrange the fractions in ascending order. Solved examples for arranging in ascending order: 1. Arrange the following fractions 5/6, 8/9, 2/3 in ascending order. First we find the L.C.M. of the denominators of the fractions to make the denominators

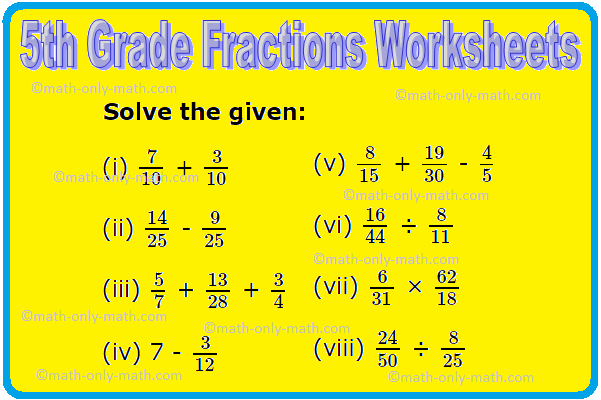
5th Grade Fractions Worksheets | Comparison of Fractions | Addition

In 5th Grade Fractions Worksheets we will solve how to compare two fractions, comparing mixed fractions, addition of like fractions, addition of unlike fractions, addition of mixed fractions, word problems on addition of fractions, subtraction of like fractions

5th Grade Fractions | Definition | Examples | Word Problems |Worksheet

In 5th Grade Fractions we will discuss about definition of fraction, concept of fractions and different types of examples on fractions. A fraction is a number representing a part of a whole. The whole may be a single object or a group of objects.

Word Problems on Fraction | Math Fraction Word Problems |Fraction Math

In word problems on fraction we will solve different types of problems on multiplication of fractional numbers and division of fractional numbers.

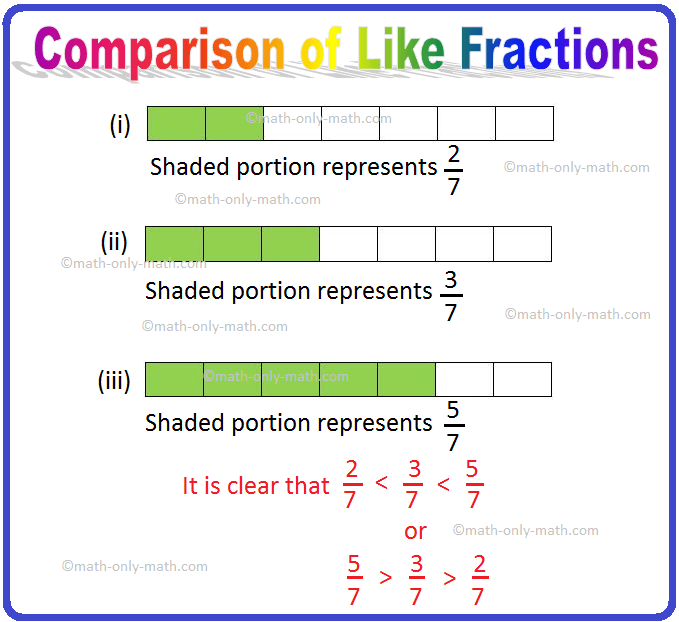
Comparison of Like Fractions | Comparing Fractions | Like Fractions

Any two like fractions can be compared by comparing their numerators. The fraction with larger numerator is greater than the fraction with smaller numerator, for example \(\frac{7}{13}\) > \(\frac{2}{13}\) because 7 > 2. In comparison of like fractions here are some

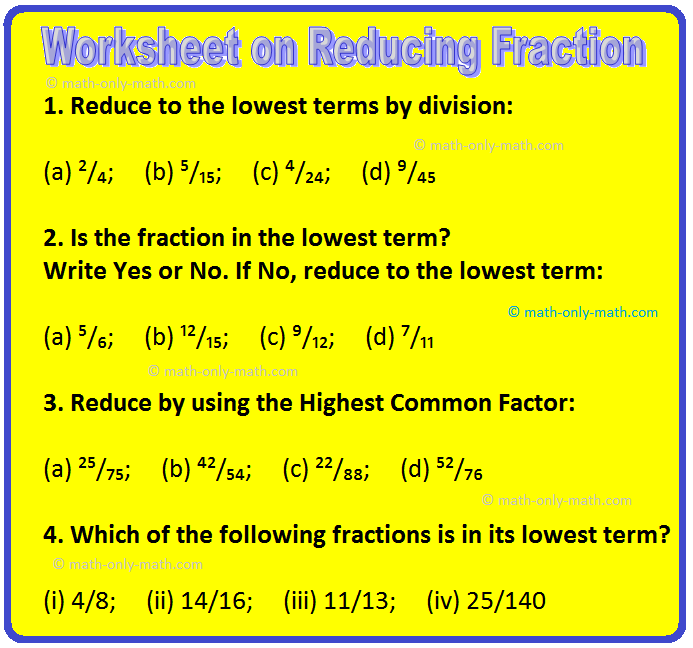
Worksheet on Reducing Fraction | Simplifying Fractions | Lowest Form

Practice the questions given in the math worksheet on reducing fraction to the lowest terms by using division. Fractional numbers are given in the questions to reduce to its lowest term.

●   Fractional Numbers - worksheets

Worksheet on Equivalent Fractions.

Worksheet on Fractions.

Worksheet on Comparison of Like Fractions.

Worksheet on Conversion of Fractions.

Worksheet on Changing Fractions.

Worksheet on Types of Fractions.

Worksheet on Reducing Fraction.

Worksheet on Addition of Fractions having the Same Denominator.

Worksheet on Subtraction of Fractions having the Same Denominator.

Worksheet on Add and Subtract Fractions.

Worksheet on Fractional Numbers.

4th Grade Math Activities

From Worksheet on Add and Subtract Fractions to HOME PAGE

Didn't find what you were looking for? Or want to know more information about Math Only Math . Use this Google Search to find what you need.

New! Comments

Share this page: What’s this?

• Preschool Activities
• Kindergarten Math
• 1st Grade Math
• 2nd Grade Math
• 3rd Grade Math
• 4th Grade Math
• 5th Grade Math
• 6th Grade Math
• 7th Grade Math
• 8th Grade Math
• 9th Grade Math
• 10th Grade Math
• 11 & 12 Grade Math
• Concepts of Sets
• Probability
• Boolean Algebra
• Math Coloring Pages
• Multiplication Table
• Cool Maths Games
• Math Flash Cards
• Online Math Quiz
• Math Puzzles
• Binary System
• Math Dictionary
• Conversion Chart
• Homework Sheets
• Math Problem Ans
• Free Math Answers
• Printable Math Sheet
• Funny Math Answers
• Employment Test
• Math Patterns
• Link Partners
• Privacy Policy

Recent Articles

Addition of Capacity | Add the Different Units of Capacity | Examples

Nov 14, 24 03:03 PM

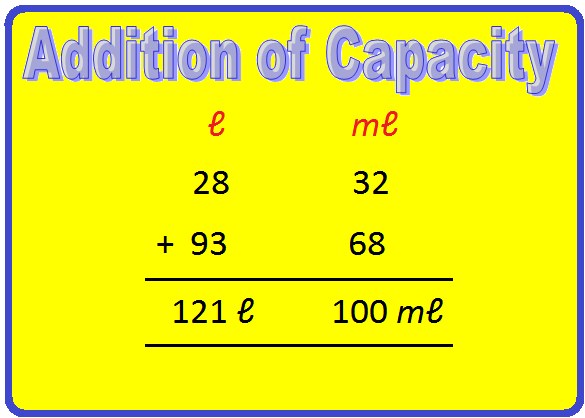
Measuring Capacity | Standard Unit of Capacity | Litre | Millilitres

Nov 14, 24 02:40 PM

Subtraction of Mass | Difference Between the Units of Mass | Examples

Nov 14, 24 09:16 AM

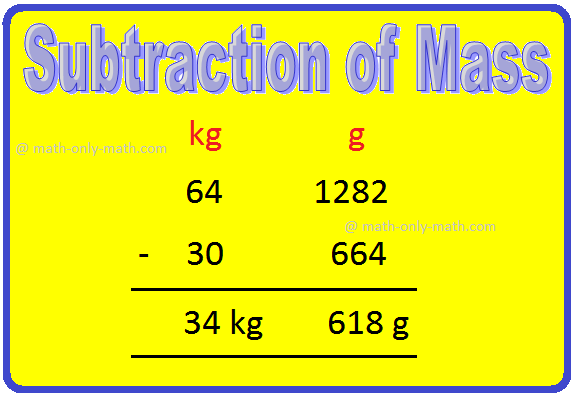
Worksheet on Subtraction of Mass |Word Problems on Subtraction of Mass

Nov 13, 24 02:00 PM

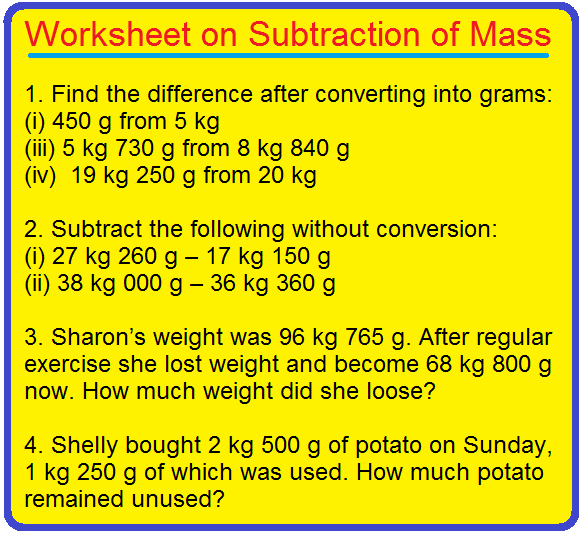
Worksheet on Addition of Mass | Word problems on Addition of Mass

Nov 13, 24 10:24 AM

© and ™ math-only-math.com. All Rights Reserved. 2010 - 2024.